Section 1.8 Quadric Surfaces II

1.8 Quadric Surfaces II

In this section, we practice more quadric surface drawing and identifying.

Common Quadratic Surfaces: Ellipsoid, Hyperboloid of One Sheet, Hyperboloid of Two Sheets, Cone, Elliptic Paraboloid, Hyperbolic Paraboloid.

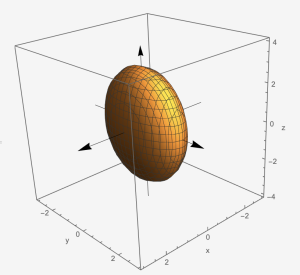
Ellipsoid: $\frac{x^2}{a^2}+\frac{y^2}{b^2}+\frac{z^2}{c^2}=1$

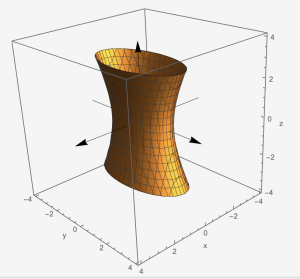
Hyperboloid of One Sheet: $\frac{x^2}{a^2}+\frac{y^2}{b^2}-\frac{z^2}{c^2}=1$

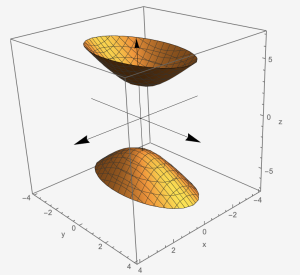
Hyperboloid of Two Sheets: $-\frac{x^2}{a^2}-\frac{y^2}{b^2}+\frac{z^2}{c^2}=1$

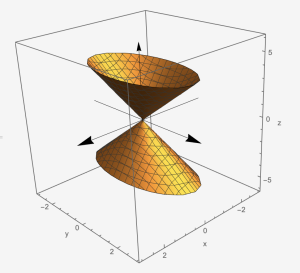
Cone: $\frac{x^2}{a^2}+\frac{y^2}{b^2}-\frac{z^2}{c^2}=0$

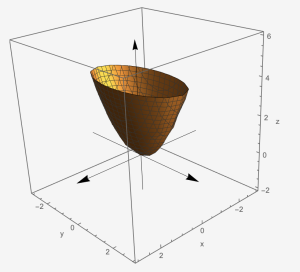
Elliptic Paraboloid: $\frac{x^2}{a^2}+\frac{y^2}{b^2}-\frac{z}{c}=0$

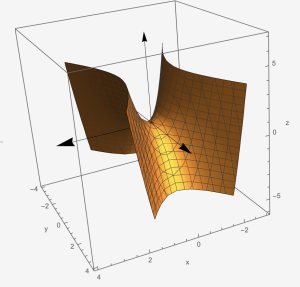
Hyperbolic Paraboloid: $\frac{x^2}{a^2}-\frac{y^2}{b^2}-\frac{z}{c}=0$

Example 1: Sketch the graph of the surface and identify the surface in the space: $x+3z=6$

Exercise 1: Sketch the graph of the surface and identify the surface in the space: $4y-z=8$.

Example 2: Sketch the graph of the surface and identify the surface in the space: $x-2y+3z=6$

Exercise 2: Sketch the graph of the surface and identify the surface in the space: $2x-4y+z=8$.

Example 3: Sketch the graph of the surface and identify the surface in the space: $y=-z^2-4$.

Exercise 3: Sketch the graph of the surface and identify the surface in the space: $x=-z^2+4$.

Example 4: Sketch the graph of the surface and identify the surface in the space: $x^2=-4y^2-z^2+16$.

Exercise 4: Sketch the graph of the surface and identify the surface in the space: $9x^2+y^2=-z^2+9$.

Example 5: Sketch the graph of the surface and identify the surface in the space: $\frac{x}{2^2}=\frac{y^2}{3^2}+\frac{z^2}{5^2}$.

Exercise 5: Sketch the graph of the surface and identify the surface in the space: $y=\frac{x^2}{3^2}+\frac{z^2}{1^2}$.

Example 6: Sketch the graph of the surface and identify the surface in the space: $\frac{x^2}{1^1}-\frac{y^2}{2^2}=\frac{z^2}{3^2}$.

Exercise 6: Sketch the graph of the surface and identify the surface in the space: $\frac{x^2}{3^2}=\frac{y^2}{1^2}-\frac{z^2}{2^2}$.

Example 7: Sketch the graph of the surface and identify the surface in the space: $-\frac{x^2}{1^2}-\frac{y^2}{2^2}+\frac{z^2}{3^2}=1$.

Exercise 7: Sketch the graph of the surface and identify the surface in the space: $\frac{x^2}{3^2}-\frac{y^2}{1^2}-\frac{z^2}{2^2}=1$.

Example 8: Sketch the graph of the surface and identify the surface in the space: $-\frac{x^2}{1^2}+\frac{y^2}{2^2}+\frac{z^2}{3^2}=1$.

Exercise 8: Sketch the graph of the surface and identify the surface in the space: $\frac{x^2}{3^2}-\frac{y^2}{1^2}+\frac{z^2}{2^2}=1$.

Example 9: Find the equation of the quadric surface with points $P(x,y,z)$ that are equidistant from point $Q(-1,0,0)$ and plane of equation $x=2$. Identify the surface.

Example 10: Sketch the region bounded by cone $x^2=y^2+z^2$ and cylinder $y^2+z^2=1$ where $0\le x\le 2$. 

Group work:

1. Find the equation of the quadric surface with points $P(x,y,z)$ that are equidistant from point $Q(0,0,-1)$ and plane of equation $z=1$. Identify the surface.

2. Determine the intersection points of parabolic hyperboloid $z=3x^2-2y^2$ with the line of parametric equations $x=3t,y=2t,z=19t,$ where $t\in R.$

3. Sketch the region bounded by elliptic paraboloid $x=y^2+z^2$ and plane $x+z=1$.

4. Sketch the region inside the paraboloid $x^2+y^2=z$ and inside the sphere $x^2+y^2+z^2=1$.

5. Sketch the region bounded by $y^2+z^2=4$, $x=0$ and $x+z=2$.