Section 3.3 Partial Derivatives

3.3 Partial Derivatives

In this section, we learn what does it mean for taking derivative of a function of two variables. Recall that the first derivative of a function $f(x)$ at $x=a$ is defined as

$$f^{\prime }(a)=\underset{x\to a}{lim}\frac{f(x)-f(a)}{x-a}=\underset{h\to 0}{lim}\frac{f(a+h)-f(a)}{h}$$

if $f(x)$ is differentiable at $x=a$. For a function of two variables, we use similar definition. The big difference is that we approach the limit at one path at a time for two dimensional space. Hence we define the derivative of a function with two variables with respect to two different directions (paths). 

Definition

Let $f(x,y)$ be a function of two variables, $x$ and $y$. The partial derivative of $f(x,y)$ with respect to $x$ at $(a,b)$ is 

$$f_x(a,b)=\underset{x\to a}{lim}\frac{f(x,b)-f(a,b)}{x-a}=\underset{h\to 0}{lim}\frac{f(a+h,b)-f(a,b)}{h}$$

if the limit exists. This definition means that we take derivative of $f(x,y)$ along the path of $y=b$. The partial derivative of $f(x,y)$ with respect to $y$ at $(a,b)$ is 

$$f_y(a,b)=\underset{y\to b}{lim}\frac{f(a,y)-f(a,b)}{y-b}=\underset{h\to 0}{lim}\frac{f(a,b+h)-f(a,b)}{h}$$

if the limit exists. This definition means that we take derivative of $f(x,y)$ along the path of $x=a$. 

Example 1: Find $f_x(1,2)$ and $f_y(1,2)$ where $f(x,y)=x^{2}y-x{y}^2+2x+y+3.$

Exercise 1: Find $f_x(2,1)$ and $f_y(2,1)$ where $f(x,y)=2x{y}^2+xy-x+2y-1.$

Recall in calculus I, if a function, $f(x)$ is differentiable over at an interval $I$, we can define the derivative function, $f^{\prime }(x)$ as 

$$f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}=\frac{\mathrm{\Delta }f}{\mathrm{△}x}.$$

We do this for a function $f(x,y)$ of two variables.

Definition: Let $f(x,y)$ be a function of two variables, $x$ and $y$. The partial derivative function of $f(x,y)$ with respect to $x$ is 

$$f_x(x,y)=\underset{h\to 0}{lim}\frac{f(x+h,y)-f(x,y)}{h}=\frac{\partial f}{\partial x}$$

if the limit exists. This definition means that we take derivative of $f(x,y)$ along the path parallel to the $x$-axis. We treat $y$-variable as a constant and we differentiate $f(x,y)$ with respect to $x$. The partial derivative function of $f(x,y)$ with respect to $y$ is 

$$f_y(x,y)=\underset{h\to 0}{lim}\frac{f(x,y+h)-f(x,y)}{h}=\frac{\partial f}{\partial y}$$

if the limit exists. This definition means that we take derivative of $f(x,y)$ along the path parallel to the $y$-axis. We treat $x$-variable as a constant and we differentiate $f(x,y)$ with respect to $y$. 

Example 2: Find $f_x(x,y)$ and $f_y(x,y)$ where $f(x,y)=x^{2}y-x{y}^2+xy+2x^2-y+2.$

Exercise 2: Find $f_x(x,y)$ and $f_y(x,y)$ where $f(x,y)=2x^{2}y+x{y}^2-3xy-2x+y^2-3.$ 

Example 3: Find $f_x(x,y)$ and $f_y(x,y)$ where $f(x,y)=(x^2+x{y}^2+y^2{)}^3.$ Then find $f_x(1,1)$.

Exercise 3: Find $f_x(x,y)$ and $f_y(x,y)$ where $f(x,y)=(x^3+x^{2}y-y^3{)}^4.$ Then find $f_y(1,-1)$.

Recall in calculus I, the first derivative of a function at $x=a$ gives the slope of tangent of the function at $x=a$. For two variable function, we have a very similar meaning. The partial derivative of $f(x,y)$ with respect to $x$ at $(x,y)=(a,b)$ is the slope of tangent of the curve(trace) that is the intersection of the surface $f(x,y)$ and $y=b$. Similarly, The partial derivative of $f(x,y)$ with respect to $y$ at $(x,y)=(a,b)$ is the slope of tangent of the curve(trace) that is the intersection of the surface $f(x,y)$ and $x=a$. 

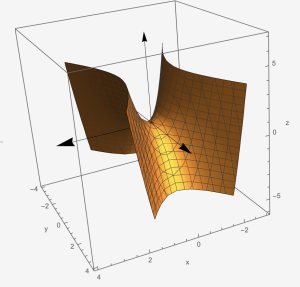
Example 4: Find $f_x(0,\pi )$ and $f_y(0,\pi )$ where $f(x,y)=\text{sin}(x^{2}y+2y).$

Exercise 4: Find $f_x(\frac{\pi }{2},0)$ and $f_y(\frac{\pi }{2},0)$ where $f(x,y)=\text{cos}(xy-2x).$

Definition: Let $f(x_1,x_2,\cdots ,x_n)$ be a function of $n$ variables. The partial derivative function of $f(x_1,\cdots ,x_n)$ with respect to $x_i$ is 

$$f_{x_i}(x_1,\cdots ,x_n)=\underset{h\to 0}{lim}\frac{f(x_1,\dots ,x_i+h,\dots ,x_n)-f(x_1,\dots ,x_i,\dots ,x_n)}{h}=\frac{\partial f}{\partial x_i}$$

if the limit exists. This definition means that we take derivative of $f(x_1,x_2,\cdots ,x_n)$ along the path parallel to the $x_i$-axis. We treat variables other than $x_i$ as constants and we differentiate $f(x_1,x_2,\cdots ,x_n)$ with respect to $x_i$. 

Example 5: Find $f_x(x,y,z)$, $f_y(x,y,z)$ and $f_z(x,y,z)$ where $f(x,y,z)=e^{x^{2}y}+yz.$

Exercise 5: Find $f_x(x,y,z)$, $f_y(x,y,z)$ and $f_z(x,y,z)$ where $f(x,y,z)=e^{xz}+xy.$

Recall in calculus I, we are able to find the second derivative of a function $f(x)$ by taking derivative of the first derivative, $f^{\prime }(x)$. At here, we have the similar approach.

Definition: Let $f(x_1,x_2,\cdots ,x_n)$ be a function of $n$ variables. The second partial derivative function of $f(x_1,\cdots ,x_n)$ with respect to $x_i$ then $x_j$ is 

$$f_{x_i{x}_j}(x_1,\cdots ,x_n)=\frac{\partial f_{x_i}}{\partial x_j}=\frac{\partial }{\partial x_j}(\frac{\partial f}{\partial x_i}).$$

Example 6: Find $f_{xy}(x,y,z)$, $f_{yx}(x,y,z)$ and $f_{yz}(x,y,z)$ where $f(x,y,z)=e^{x^{2}y}+yz.$

Exercise 6: Find $f_{xy}(x,y,z)$, $f_{yx}(x,y,z)$ and $f_{xz}(x,y,z)$ where $f(x,y,z)=e^{xz}+xy.$

Theorem:(Clairaut’s Theorem)

Suppose that $f(x,y)$ is defined on an open disk $D$ that contains the point $(a,b)$. If the functions $f_{xy}$ and $f_{yx}$ are continuous on $D$, then $f_{xy}=f_{yx}$.

Remark: Higher partial derivative can also be defined and we have $f_{xyz}=f_{xzy}=f_{yxz}=f_{zyx}=f_{yzx}=f_{zxy}.$

Example 7: Find $f_{yzx}(x,y,z)$, and $f_{yyx}(x,y,z)$ where $f(x,y,z)=e^x+\text{tan}(yz).$

Exercise 7: Find $f_{xyz}(x,y,z)$, and $f_{yzz}(x,y,z)$ where $f(x,y,z)=e^z+\text{sin}(xy).$

Example 8: Find $f_y(x,y)$, and $f_x(x,y)$ where $f(x,y)=y^x.$

Example 9: Use implicit differentiation to find $\frac{\partial z}{\partial x}$ and $\frac{\partial z}{\partial y}$ where $e^z=xyz$.

Group work:

1. Find $f_y(x,y)$, and $f_x(x,y)$ where $f(x,y)=x^y.$

2. Use implicit differentiation to find $\frac{\partial z}{\partial x}$ and $\frac{\partial z}{\partial y}$ where $\text{sin}(x)=xy{z}^2$.

3. Find $f_{yzx}(x,y,z)$, and $f_{yyx}(x,y,z)$ where $f(x,y,z)=e^x+\text{tan}(yz).$

4. Find $f_{xyz}(x,y,z)$, and $f_{yzz}(x,y,z)$ where $f(x,y,z)=z^{2}yx+{\text{sin}}^{-1}(xy).$

5. Use implicit differentiation to find $\frac{\partial z}{\partial x}$ and $\frac{\partial z}{\partial y}$ where $e^{yz}=\text{cos}(xy)$.