Section 3.4 Tangent Planes

3.4 Tangent Planes

In this section, we learn the equation of a tangent plane at a given point on a surface. Recall in calculus I, given a function $y=f(x)$ and $x=x_0$, we can find the equation of the tangent line at $x=x_0$ if $f(x)$ is differentiable at $x=x_0$. The equation is 

$$\frac{y-y_0}{x-x_0}=f^{\prime }(x_0).$$

We can rewrite the equation as 

$$y-y_0=f^{\prime }(x_0)(x-x_0)$$

$$y=y_0+f^{\prime }(x_0)(x-x_0).$$

Keep in mind that $f^{\prime }(x_0)$ is the slope of the tangent at $x=x_0$, hence in a 2-dimensional space, the direction of the line is $(1,f^{\prime }(x_0))$. This is because $f^{\prime }(x_0)$ is the rate of change of the function with respect to the $x$-direction, or every time we move one unit to the right of $x_0$, the $f(x)$ value move $f^{\prime }(x_0)$ unit from $f(x_0)$. We use similar idea for the 3-dimensional space. We define the tangent plane first.

Definition

Let $P_0=(x_0,y_0,z_0)$ be a point on a surface $S$, and let $C$ be any curve passing through $P_0$ and lying entirely in $S$. If the tangent lines to all such curves $C$ at $P_0$ lie in the same plane, then this plane is called the tangent plane to $S$ at $P_0$.

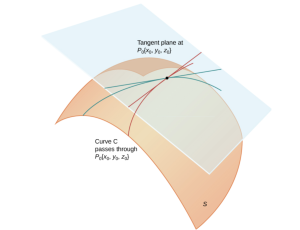
In order to find the equation of the tangent plane, we need two directions on the plane in order to find so call the tangent plane of $f(x,y)$ at $(x_0,y_0)$. 

Definition

Let $S$ be a surface defined by a differentiable function $z=f(x,y)$, and let $P_0=(x_0,y_0)$ be a point in the domain of $f$. Then, the equation of the tangent plane to $S$ at $P_0$ is given by

$$z=f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0).$$

Example 1: Find the equation of the tangent plane to the surface defined by the function $f(x,y)=x^{2}y-x{y}^2+2x+y+3$ at point $(2,-1)$.

Exercise 1: Find the equation of the tangent plane to the surface defined by the function $f(x,y)=2x{y}^2+xy-x+2y-1$ at $(1,2)$.

Recall in calculus I, if a function, $f(x)$ is differentiable over at $x=x_0$, we cal $L(x)=y_0+f^{\prime }(x_0)(x-x_0)$ the linearization of $f(x)$ at $x=x_0$. We have the similar definition for surface.

Definition:

Given a function $z=f(x,y)$ with continuous partial derivatives that exist at the point $(x_0,y_0)$, the linear approximation of $f$ at the point $(x_0,y_0)$ is given by the equation

$$L(x,y)=f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0).$$

Example 2: Given the function $f(x,y)=\sqrt[3]{33-2x^2-y^2}$, approximate $f(1.1,1.9)$ using the point $(1,2)$. 

Exercise 2: Given the function $f(x,y)=\sqrt{7+x^2-y^2}$, approximate $f(2.9,0.1)$ using the point $(3,0)$. 

Definition: 

A function $f(x,y)$ is differentiable at a point $P_0=(x_0,y_0)$ if, for all points $(x,y)$ in a $\delta$ disk around $P_0$, we can write 

$$f(x,y)=f(x_0,y_0)+f_x(x_0,y_0)(x-x_0)+f_y(x_0,y_0)(y-y_0)+E(x,y).$$

where the error term $E(x,y)$ satisfies 

$$\underset{(x,y)\to (x_0,y_0)}{lim}\frac{E(x,y)}{\sqrt{(x-x_0{)}^2+(y-y_0{)}^2}}=0.$$

Remark: In general, testing if a function is differentiable is not trivial because the difficulty of the computation of the limit for two variables. 

$$f(x,y)=\{\begin{array}{ll}\frac{xy}{x^2+y^2}& (x,y)\ne (0,0)\\ 0& (x,y)=(0,0)\end{array}$$

 is not differentiable at $(0,0)$. 

Theorem: Differentiability Implies Continuity 

Let $z=f(x,y)$ be a function of two variables with $(x_0,y_0)$ in the domain of $f$ . If $f(x,y)$ is differentiable at $(x_0,y_0)$, then $f(x,y)$ is continuous at $(x_0,y_0)$.

Theorem: Continuity of First Partials Implies Differentiability 

Let $z=f(x,y)$ be a function of two variables with $(x_0,y_0)$ in the domain of f. If $f(x,y),f_x(x,y)$, and $f_y(x,y)$ all exist in a neighborhood of $(x_0,y_0)$ and are continuous at

$(x_0,y_0)$, then $f(x,y)$ is differentiable there. 

Definition:

Let $z=f(x,y)$ be a function of two variables with $(x_0,y_0)$ in the domain of $f$, and let $\mathrm{\Delta }x$ and $\mathrm{\Delta }y$ be chosen so that $(x_0+\mathrm{\Delta }x,y_0+\mathrm{\Delta }y)$ is also in the domain of $f$. If $f$ is differentiable at the point $(x_0,y_0)$, then the differentials $dx$ and $dy$ are defined as $dx=\mathrm{\Delta }x$ and $dy=\mathrm{\Delta }y$. The differential $dz$, also called the total differential of $z=f(x,y)$ at $(x_0,y_0)$, is defined as  $$dz=f_x(x_0,y_0)dx+f_y(x_0,y_0)dy.$$

Example 3: Find the differential $dz$ of the function $f(x,y)=x^2+3xy-y^2$ and use it to approximate $\mathrm{\Delta }z$ at point $(1,2)$. Use $\mathrm{\Delta }x=0.1$ and $\mathrm{\Delta }y=-0.05.$ What is the exact value of $\mathrm{\Delta }z$?

Exercise 3: Find the differential $dz$ of the function $f(x,y)=2x^2-2xy+y^2$ and use it to approximate $\mathrm{\Delta }z$ at point $(2,-1)$. Use $\mathrm{\Delta }x=-0.01$ and $\mathrm{\Delta }y=0.1$. What is the exact value of $\mathrm{\Delta }z$?

Example 4: The base radius and height of a right circular cone are measured as $10$ in. and $25$ in., respectively, with a possible error in measurement of as much as $0.1$ in. each. Use differentials to estimate the maximum error in the calculated volume of the cone. $V=\frac{1}{3}\pi r^{2}h$.

Exercise 4: The base radius and height of cylindrical can are measured as $5$ in. and $10$ in., respectively, with a possible error in measurement of as much as $0.01$ in. each. Use differentials to estimate the maximum error in the calculated volume of the can. $V=\pi r^{2}h$.

Example 5: Given the function $f(x,y,z)=\text{sin}(x)+\sqrt{y^2+z^2}$, approximate $f(0.1,0.9,-0.1)$ using the point $(0,1,0)$.

Exercise 5: Given the function $f(x,y,z)=\sqrt{x^2+y^2+z^2}$, approximate $f(0.9,0.1,2.1)$ using $(1,0,2)$.

Example 6: Find the equation of the tangent plane to the surface defined by the function $z=f(x,y)=\text{ln}(10x^2+2y^2+1)$ at point $(0,0,0)$.

Example 7: Find the linear approximation of the function $f(x,y)=e^x\text{cos}(y)$

at $P(0,0)$.

Group work:

1. Find the equation of the tangent plane to the surface defined by the function $f(x,y)=\text{arctan}(x+2y)$ at point $(1,0)$.

2. Find the linear approximation of the function $f(x,y)=x\sqrt{y}$ at $P(1,4)$.

3. Electrical power $P$ is given by $P=\frac{V^2}{R}$ , where $V$ is the voltage and $R$ is the resistance. Approximate the maximum error in calculating power if $240$V is applied to a $1000W$ resistor and the possible  errors in measuring V and R are $0.02$ and $0.03$, respectively.

4. Find the total differential of the function $w=e^y\text{cos}(x)+z^2.$