Section 4.1 Double Integrals

4.1 Double Integrals

In calculus I, we learn one of most important reasons for learning calculus, finding areas. We use Riemann sum to define an integral:

let $f(x)$ be a function defined over the interval, $[a,b]$ and we divide the interval into $n$-subintervals, $[x_{i-1},x_i]$ where $i=1,...,n$, $x_0=a$ , and $x_n=b$. We choose $x_i^{\ast }$ inside $[x_{i-1},x_i]$, then

$${\int }_a^{b}f(x)dx=\underset{n\to \mathrm{\infty }}{lim}\sum _{i=1}^{n}f(x_i^{\ast })\mathrm{△}x$$

 where $\mathrm{△}x=\frac{b-a}{n}$ the length of the interval $[x_{i-1},x_i]$ (under the assumption that the limit exists). If $f(x)\ge 0$ then ${\int }_a^{b}f(x)dx$ present the area between $f(x)$ and $x$-axis from $x=a$ to $x=b$. The idea is built from adding up rectangles with width $\mathrm{△}x=\frac{b-a}{n}$ and height $f(x_i^{\ast })$ at the interval $[x_{i-1},x_i]$. 

For two variables functions, we have similar definitions and approach. If $f(x,y)$ is a function with two variables defined over $R=[a,b]\times [c,d]$, suppose $f(x,y)\ge 0$ for any $(x,y)$ in $R$. Then we can find the volume under the surface $f(x,y)$ above the $xy$-plane using Riemann sum. First we divide $[a,b]$ into $m$-subintervals and $[c,d]$ into $n$-subintervals and we have the $ij$-th rectangle $[x_{i-1},x_i]\times [y_{j-1},y_j]$. We choose $(x_i^{\ast },y_j^{\ast })$ inside $[x_{i-1},x_i]\times [y_{j-1},y_j]$,

then $$\underset{(m,n)\to (\mathrm{\infty },\mathrm{\infty })}{lim}\sum _{j=1}^n\sum _{i=1}^{m}f(x_i^{\ast },y_j^{\ast })\mathrm{△}x\mathrm{△}y$$ presents the volume under the surface $f(x,y)$ above the $xy$-plane ( if the limit exists) where $\mathrm{△}x=\frac{b-a}{m}$ and $\mathrm{△}y=\frac{d-c}{n}$. 

Definition: Double integral

Let $f(x,y)$ be a function defined over $R$. The double integral of the function $f(x,y)$ over the rectangular region $R$ in the $xy$-plane is defined as

$${\iint }_{R}f(x,y)dA=\underset{(m,n)\to (\mathrm{\infty },\mathrm{\infty })}{lim}\sum _{j=1}^n\sum _{i=1}^{m}f(x_i^{\ast },y_j^{\ast })\mathrm{△}A.$$

Remark:

If $f(x,y)\ge 0$, then the volume $V$ of the solid $S$, which lies above $R$ in the $xy$-plane and under the graph of $f$, is the double integral of the function $f(x,y)$ over the rectangle $R$. If the function is ever negative, then the double integral can be considered a \textquotedblleft signed\textquotedblright{} volume in a manner similar to the way we defined net signed area.

Definition 

Assume $a,b,c,$ and $d$ are real numbers. We define an iterated integral for a function $f(x,y)$ over the rectangular region $R=[a,b]\times [c,d]$ as 

$${\int }_c^d{\int }_a^{b}f(x,y)dxdy={\int }_c^d[{\int }_a^{b}f(x,y)dx]dy={\int }_c^d[A(y)]dy$$

$${\int }_a^b{\int }_c^{d}f(x,y)dydx={\int }_a^b[{\int }_c^{d}f(x,y)dy]dx={\int }_a^b[B(x)]dx.$$

Theorem: Fubini’s Theorem 

Suppose that $f(x,y)$ is a function of two variables that is continuous over a rectangular region $R=[a,b]\times [c,d]$. Then the double integral of $f$ over the region equals an iterated integral, 

$${\iint }_{R}f(x,y)dA={\int }_c^d{\int }_a^{b}f(x,y)dxdy={\int }_a^b{\int }_c^{d}f(x,y)dydx$$

More generally, Fubini\textquoteright s theorem is true if $f$ is bounded on $R$ and $f$ is discontinuous only on a finite number of continuous curves. In other words, $f$ has to be integrable over $R$.

Example 1: Compute the double integral ${\iint }_{R}f(x,y)dA$ where $f(x,y)=x$ and $R=[0,2]\times [-1,1]$.

Exercise 1: Compute the double integral ${\iint }_{R}f(x,y)dA$ where $f(x,y)=y$ and $R=[-2,0]\times [0,3]$.

Example 2: Compute the double integral ${\int }_{-2}^0{\int }_0^2(x+xy{)}^{2}dxdy$.

Exercise 2: Compute the double integral${\int }_0^3{\int }_{-1}^0(y-xy{)}^{2}dxdy$.

Remark:

$${\iint }_{R}f(x)g(y)dxdy={\int }_a^{b}f(x)dx{\int }_c^{d}g(y)dy.$$

Example 3: Compute the double integral ${\int }_1^3{\int }_0^2{x}^{2}y\text{ln}ydxdy$.

Exercise 3: Compute the double integral ${\int }_1^3{\int }_{-1}^{3}x{e}^{x}ydxdy$.

Definition 

The average value of a function of two variables over a region $R$ is 

$$f_{\text{avg}}=\frac{1}{\text{area}R}{\iint }_{R}f(x,y)dA.$$

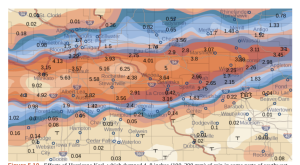
Example 4: The weather map below shows an unusually moist storm system associated with the remnants of Hurricane Karl, which dumped 4-8 inches (100-200 mm) of rain in some parts of the Midwest on September 22-23, 2010. The area of rainfall measured 300 miles east to west and 250 miles north to south. Estimate the average rainfall over the entire area in those two days.

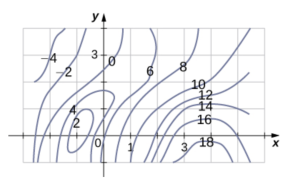
Exercise 4: A contour map is shown for a function $f(x,y)$ on the rectangle $R=[-3,6]\times [-1,4].$

a. Use the midpoint rule with $m=3$ and $n=2$ to estimate the value of ${\iint }_{R}f(x,y)dA.$. 

b. Estimate the average value of the function $f(x,y)$.

Example 5: Compute the double integral ${\int }_0^2{\int }_1^{2}x{e}^{x-y}dxdy$.

Exercise 5: Compute the double integral ${\int }_1^2{\int }_0^{2}y{e}^{y-x}dxdy$. 

Example 6: Find the volume of the solid enclosed by the surfaces, $z=2x^2+x{y}^2$ and the planes, $z=0$, $y=\pm 2$, $x=0$ and $x=2$.

Example 7: Find the volume of the solid in the first octant bounded by $z=9-y^2$ and the plane $x=5$.

Group work:

1. Find the volume of the solid enclosed by the surfaces, $z=x+x^{2}y$ and the planes, $z=0$, $x=\pm 1$, $y=0$ and $y=2$.

2. Find the volume of the solid in the first octant bounded by $z=16-x^2$ and the plane $y=5$.

3. Compute the double integral ${\int }_0^3{\int }_1^2\frac{x}{x^2+y^2}dxdy$.

4. Compute the double integral ${\int }_{-1}^2{\int }_1^3\frac{y}{x^2+y^2}dxdy$.