2.6 Rate of Change and The Derivative
Rate of Change and the Derivative
As we introduce the concept of a derivative of a function, we will see that this has links to familiar notions from algebra such as slope and rate of change.
We are often interested in measuring rates of change such as the change in a population, change in concentration of a drug in the bloodstream, change in revenue for a company, change in velocity of a car, etc.
The following example allows us to investigate the rate of change in velocity for a falling object.
Instantaneous Velocity
Suppose we drop a tomato from the top of a 100 foot building and time its fall.
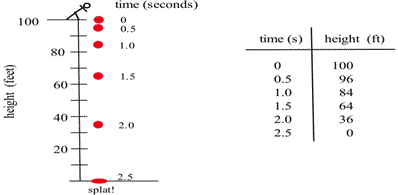
Long description: Each dot has a time measurement in seconds, respectively as 0, 0.5, 1.0, 1.5, 2 and 2.5.
Some questions are easy to answer directly from the table:
- How long did it take for the tomato to drop 100 feet? (2.5 seconds)
- How far did the tomato fall during the first second? (100 – 84 = 16 feet)
- How far did the tomato fall during the last second? (64 – 0 = 64 feet)
- How far did the tomato fall between
Some questions require a little calculation:
- What was the average velocity of the tomato during its fall?
- What was the average velocity between
Some questions are more difficult:
- How fast was the tomato falling 1 second after it was dropped?This question is significantly different from the previous two questions about average velocity. Here we want the instantaneous velocity, the velocity at an instant in time. Unfortunately the tomato is not equipped with a speedometer so we will have to give an approximate answer.One crude approximation of the instantaneous velocity after 1 second is simply the average velocity during the entire fall, -40 ft/s . But the tomato fell slowly at the beginning and rapidly near the end so the “-40 ft/s” estimate may or may not be a good answer.We can get a better approximation of the instantaneous velocity at
In general, the shorter the time interval over which we calculate the average velocity, the better the average velocity will approximate the instantaneous velocity. The average velocity over a time interval is

Long description: The horizontal axis is labeled as time in seconds. The vertical axis is labeled as height in feet. A line is tangent to the curve at (0.5, 96) with the label m = -24 ft/s. A dashed tangent line is shown at the point (1.0, 84) with the label m = -40 ft/s.
Average vs Instantaneous Velocity
Average velocity =
Instantaneous velocity = slope of the line tangent to the graph.
Growing Bacteria
Suppose we set up a machine to count the number of bacteria growing on a Petri plate. At first there are few bacteria so the population grows slowly. Then there are more bacteria to divide so the population grows more quickly. Later, there are more bacteria and less room and nutrients available for the expanding population, so the population grows slowly again. Finally, the bacteria have used up most of the nutrients, and the population declines as bacteria die.
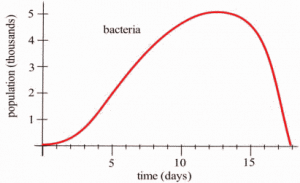
Long description: The peak of the curve occurs at approximately (13, 5). The curve is labeled as bacteria. The horizontal axis extends from 0 to 20 with label time in days. The vertical axis extends from 0 to 5 with the label population in thousands.
The population graph can be used to answer a number of questions.
- What is the bacteria population at time
- What is the population increment from
- What is the rate of population growth from
- What is the rate of population growth on the third day, at
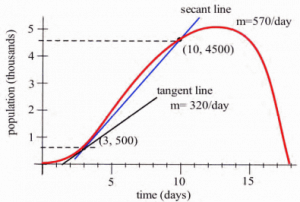
Long description: The peak of the curve occurs at approximately (13, 5). The curve is labeled as bacteria. The horizontal axis extends from 0 to 20 with label time in days. The vertical axis extends from 0 to 5 with the label population in thousands. A tangent line is shown passing through (3, 500) with the label tangent line m = 320/day. A secant line passes through the points (3, 500) and (10, 4500) with the label secant line m = 570/day.
Tangent Lines
Try this!
The graph below is the graph of
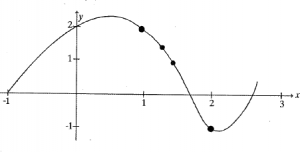
Long description: The graph begins at (1, 0) and increases to a maximum at (0.8, 2.1). Then the graph decreases and reaches a minimum at (2, -1) and then the graph increases until the point (2.6, 0.3).
First, draw the secant line between (1, 2) and (2, −1) and compute its slope. Note: A secant line is a straight line connecting two points on a curve.Now draw the secant line between (1, 2) and (1.5, 1) and compute its slope.
Compare the two lines you have drawn. Which would be a better approximation of the tangent line to the curve at (1, 2)?
Now draw the secant line between (1, 2) and (1.3, 1.5) and compute its slope. Is this line an even better approximation of the tangent line?
Now draw your best guess for the tangent line and measure its slope. Do you see a pattern in the slopes?
You should have noticed that as the interval got smaller and smaller, the secant line got closer to the tangent line and its slope got closer to the slope of the tangent line. That’s good news–we know how to find the slope of a secant line.
In some applications, we need to know where the graph of a function
Example 1
Below is the graph of
Long description: The graph decreases then increases then decreases then increases then decreases.
The tangent lines to the graph of
Let’s explore further this idea of finding the tangent slope based on the secant slope.
Example 2
Find the slope of the line
We could estimate the slope of
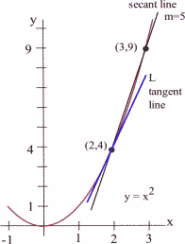
Long description: The x-axis extends from -1 to 3 and the y-axis extends from 0 to 10. A tangent line is shown passing through (2, 4). A secant line is shown passing through (2, 4) and (3, 9) with label m = 5.
We can see that the line through (2,4) and (3,9) on the graph of
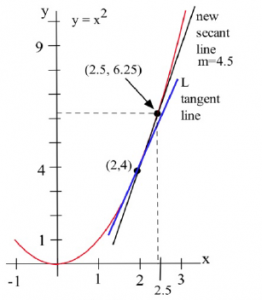
From the second figure, we can see that the slope of the line through the points (2,4) and (2.5,6.25) is a better approximation of the slope of the tangent line at (2,4):
| Slope of line through |
||
|---|---|---|
| 1.5 | 2.25 | 3.5 |
| 1.9 | 3.61 | 3.9 |
| 1.99 | 3.9601 | 3.99 |
| Slope of line through |
||
|---|---|---|
| 3 | 9 | 5 |
| 2.5 | 6.25 | 4.5 |
| 2.01 | 4.0401 | 4.01 |
The only thing special about the x–values we picked is that they are numbers which are close, and very close, to
We can bypass much of the calculating by not picking the points one at a time: let’s look at a general point near (2,4). Define
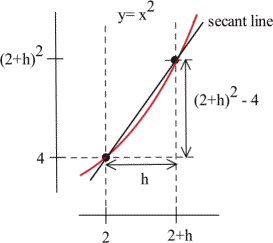
Long description: The horizontal axis is shown as extending from 2 to 2 + h. The vertical axis is shown extending from 4 to (2 + h)^2. A secant line is labeled and passes through the points (2, 4) and (2+h, (2+h)^2).
We can easily evaluate this limit using direct substitution, finding that as the interval
The tangent line problem and the instantaneous velocity problem are the same problem. In each problem we wanted to know how rapidly something was changing at an instant in time, and the answer turned out to be finding the slope of a tangent line, which we approximated with the slope of a secant line. This idea is the key to defining the slope of a curve.
The Derivative
We can view the derivative in different ways. Here are a three of them:
- The derivative of a function
- The derivative is the slope of the tangent line to the graph of
- The derivative is the slope of the curve
A function is called differentiable at
Notation for the Derivative
The derivative of
The notation that resembles a fraction is called Leibniz notation. It displays not only the name of the function (
Verb Forms
We find the derivative of a function, or take the derivative of a function, or differentiate a function.
We use an adaptation of the
Formal Algebraic Definition
Practical Definition
The derivative can be approximated by looking at an average rate of change, or the slope of a secant line, over a very tiny interval. The tinier the interval, the closer this is to the true instantaneous rate of change, slope of the tangent line, or slope of the curve.
Looking Ahead
We will have methods for computing exact values of derivatives from formulas soon. If the function is given to you as a table or graph, you will still need to approximate this way.
This is the foundation for our discussion of derivatives. It’s remarkable that such a simple idea (the slope of a tangent line) and such a simple definition (for the derivative
Example 3
Find the slope of the tangent line to
The slope of the tangent line is the value of the derivative
We can simplify by giving the fractions a common denominator:
Thus, the slope of the tangent line to
The Derivative as a Function
We now know how to find (or at least approximate) the derivative of a function for any
Example 4
Below is the graph of a function
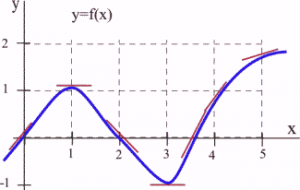
Long description: The x-axis extends from 0 to 5. The y-axis extends from 0 to 2. A maximum is reached at (1,1) and a minimum is reached at (3, -1).
At various values of
| 0 | 0 | 1 |
|---|---|---|
| 1 | 1 | 0 |
| 2 | 0 | -1 |
| 3 | -1 | 0 |
| 3.5 | 0 | 2 |
We can estimate the values of
We can even think about entire intervals. For example, if
The values of

Long description: The x-axis extends from 0 to 5 and the y-axis extends from -1 to 2. The label on the graph is m(x) = slope of f(x).
Example 5
Shown is the graph of the height
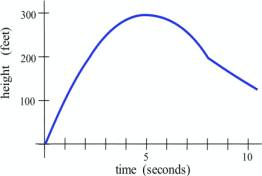
Long description: The x-axis extends from 0 to 10 and is labeled as time in seconds. The vertical axis extends from 0 to 300 and is labeled as height in feet. The graph starts at (0, 0) and reaches a maximum at (5, 300) and then decreases.
Sketch the graph of the velocity of the rocket at time
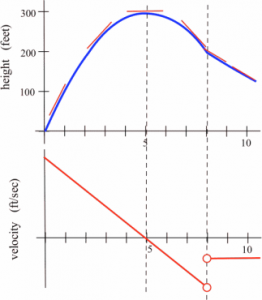
Long description: The top graph is shown that increases then decreases. The x-axis extends from 0 to 10. The vertical axis extends from 0 to 300 and is labeled as height in feet. The graph starts at (0, 0) and reaches a maximum at (5, 300) and then decreases. The bottom graph shows a decreasing line which passes through (5, 0). The vertical axis is labeled as velocity in ft/sec.
We can also find derivative functions algebraically using limits.
Example 6
Find
Setting up the derivative using a limit,
We will start by simplifying
Now finding the limit:
We can find the limit of this expression by direct substitution:
Notice that the derivative depends on
A formula for the derivative function is very powerful, but as you can see, calculating the derivative using the limit definition is very time consuming. In the next section, we will identify some patterns that will allow us to start building a set of rules for finding derivatives without needing the limit definition.
Interpreting the Derivative
So far we have emphasized the derivative as the slope of the line tangent to a graph. That interpretation is very visual and useful when examining the graph of a function, and we will continue to use it. Derivatives, however, are used in a wide variety of fields and applications, and some of these fields use other interpretations. The following are a few interpretations of the derivative that are commonly used.
General
Rate of Change:
Graphical
Slope:
Physical
Velocity: If
Acceleration: If
Business
Marginal Cost, Marginal Revenue, and Marginal Profit: We’ll explore these terms in more depth later in the section. Basically, the marginal cost is approximately the additional cost of making one more object once we have already made
In business contexts, the word “marginal” usually means the derivative or rate of change of some quantity.
One of the strengths of calculus is that it provides a unity and economy of ideas among diverse applications. The vocabulary and problems may be different, but the ideas and even the notations of calculus are still useful.
Example 7
Suppose the demand curve for widgets was given by
Note that we calculated
Since thousands of widgets
and the units for
Specifically,

