6.6 Area Between Two Curves

Area of a Region between Two Curves

Let $f(x)$ and $g(x)$ be continuous functions over an interval $[a,b]$ such that $f(x)\ge g(x)$ on $[a,b].$ We want to find the area between the graphs of the functions, as shown in the following figure.

Long description: There are two curves on the graph. The higher curve is labeled “f(x)” and the lower curve is labeled “g(x)”. There are two boundaries on the x-axis labeled a and b. There is shaded area between the two curves bounded by lines at x=a and x=b.

As we did before, we are going to partition the interval on the $x\text{-axis}$ and approximate the area between the graphs of the functions with rectangles. So, for $i=0,1,2\text{,…},n,$ let $P=\left\{x_i\right\}$ be a regular partition of $[a,b].$ Then, for $i=1,2\text{,…},n,$ choose a point $x_i^{\ast }\in [x_{i-1},x_i],$ and on each interval $[x_{i-1},x_i]$ construct a rectangle that extends vertically from $g(x_i^{\ast })$ to $f(x_i^{\ast }).$ (Figure)(a) shows the rectangles when $x_i^{\ast }$ is selected to be the left endpoint of the interval and $n=10.$ (Figure)(b) shows a representative rectangle in detail.

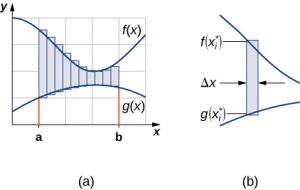
Long description: The higher curve is labeled “f(x)” and the lower curve is labeled “g(x)”. There are two boundaries on the x-axis labeled a and b. There is shaded area between the two curves bounded by lines at x=a and x=b. The second graph, labeled “(b)” has two curves one over the other. The first curve is labeled “f(x*)” and the lower curve is labeled “g(x*)”. There is a shaded rectangle between the two. The width of the rectangle is labeled as “delta x”.

The height of each individual rectangle is $f(x_i^{\ast })-g(x_i^{\ast })$ and the width of each rectangle is $\text{Δ}x.$ Adding the areas of all the rectangles, we see that the area between the curves is approximated by

$$A\approx \underset{i=1}{\text{∑}^n}[f(x_i^{\ast })-g(x_i^{\ast })]\text{Δ}x.$$

This is a Riemann sum, so we take the limit as $n\to \mathrm{\infty }$ and we get

$$A=\underset{n\to \mathrm{\infty }}{\text{lim}}\underset{i=1}{\text{∑}^n}[f(x_i^{\ast })-g(x_i^{\ast })]\text{Δ}x={\int }_a^b[f(x)-g(x)]dx.$$

These findings are summarized in the following theorem.

 

We apply this theorem in the following example.

 

In the example above, we defined the interval of interest as part of the problem statement. Quite often, though, we want to define our interval of interest based on where the graphs of the two functions intersect. This is illustrated in the following example.

 

Areas of Compound Regions

So far, we have required $f(x)\ge g(x)$ over the entire interval of interest, but what if we want to look at regions bounded by the graphs of functions that cross one another? In that case, we modify the process we just developed by using the absolute value function.

 

In practice, applying this theorem requires us to break up the interval $[a,b]$ and evaluate several integrals, depending on which of the function values is greater over a given part of the interval. We study this process in the following example.

 

Regions Defined with Respect to $y$

In our previous slides, we had to evaluate two separate integrals to calculate the area of the region. However, there is another approach that requires only one integral. What if we treat the curves as functions of $y,$ instead of as functions of $x?$ Review (Figure). Note that the left graph, shown in red, is represented by the function $y=f(x)=x^2.$ We could just as easily solve this for $x$ and represent the curve by the function $x=v(y)=\sqrt{y}.$ (Note that $x=\text{−}\sqrt{y}$ is also a valid representation of the function $y=f(x)=x^2$ as a function of $y.$ However, based on the graph, it is clear we are interested in the positive square root.) Similarly, the right graph is represented by the function $y=g(x)=2-x,$ but could just as easily be represented by the function $x=u(y)=2-y.$ When the graphs are represented as functions of $y,$ we see the region is bounded on the left by the graph of one function and on the right by the graph of the other function. Therefore, if we integrate with respect to $y,$ we need to evaluate one integral only. Let’s develop a formula for this type of integration.

Let $u(y)$ and $v(y)$ be continuous functions over an interval $[c,d]$ such that $u(y)\ge v(y)$ for all $y\in [c,d].$ We want to find the area between the graphs of the functions, as shown in the following figure.

Long description: They are the functions v(y) and u(y). In between these graphs is a shaded region, bounded to the left by v(y) and to the right by u(y). The region is labeled R. The shaded area is between the horizontal boundaries of y=c and y=d.

This time, we are going to partition the interval on the $y\text{-axis}$ and use horizontal rectangles to approximate the area between the functions. So, for $i=0,1,2\text{,…},n,$ let $Q=\left\{y_i\right\}$ be a regular partition of $[c,d].$ Then, for $i=1,2\text{,…},n,$ choose a point $y_i^{\ast }\in [y_{i-1},y_i],$ then over each interval $[y_{i-1},y_i]$ construct a rectangle that extends horizontally from $v(y_i^{\ast })$ to $u(y_i^{\ast }).$ (Figure)(a) shows the rectangles when $y_i^{\ast }$ is selected to be the lower endpoint of the interval and $n=10.$ (Figure)(b) shows a representative rectangle in detail.

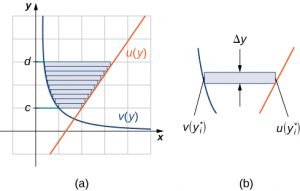
Long description: The first figure has two curves. They are the functions v(y*) and u(y*). In between these curves is a horizontal rectangle. The second figure labeled “(a)”, is a shaded region, bounded to the left by v(y) and to the right by u(y). The shaded area is between the horizontal boundaries of y=c and y=d. This shaded area is broken into rectangles between the curves. The third figure, labeled “(b)”, is the two curves v(y*) and u(y*). In between the curves is a horizontal rectangle with width delta y.

The height of each individual rectangle is $\text{Δ}y$ and the width of each rectangle is $u(y_i^{\ast })-v(y_i^{\ast }).$ Therefore, the area between the curves is approximately

$$A\approx \underset{i=1}{\text{∑}^n}[u(y_i^{\ast })-v(y_i^{\ast })]\text{Δ}y.$$

This is a Riemann sum, so we take the limit as $n\to \mathrm{\infty },$ obtaining

$$A=\underset{n\to \mathrm{\infty }}{\text{lim}}\underset{i=1}{\text{∑}^n}[u(y_i^{\ast })-v(y_i^{\ast })]\text{Δ}y={\int }_c^d[u(y)-v(y)]dy.$$

These findings are summarized in the following theorem.