Facts About the Chi-Square Distribution

The notation for the chi-square distribution is $displaystylechisimch{i}_{df}^2$, where df = degrees of freedom which depends on how chi-square is being used. (If you want to practice calculating chi-square probabilities then use $displaystyledf=n-1$. The degrees of freedom for the three major uses are each calculated differently.)

For the χ² distribution, the population mean is μ = df and the population standard deviation is $displaystylesigm{a}_{ch{i}^2}=sqrt2(df)$.

The random variable is shown as χ², but may be any upper case letter.

The random variable for a chi-square distribution with k degrees of freedom is the sum of k independent, squared standard normal variables.

$displaystylech{i}^2=(Z_1{)}^2+(Z_2{)}^2+dots+(Z_k{)}^2$

1. The curve is nonsymmetrical and skewed to the right.
2. There is a different chi-square curve for each df.
   
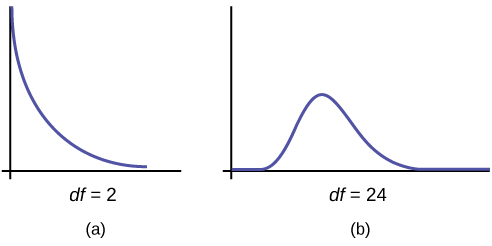
3. The test statistic for any test is always greater than or equal to zero.
4. When df > 90, the chi-square curve approximates the normal distribution. For $displaystyleXsimch{i}_{1,000}^2$ the mean, $displaystylemu=df=1,000$ and the standard deviation, $displaystylesigma=sqrt2(1,000)$. Therefore, $displaystyleXsimN(1,000,44.7)$, approximately.
5. The mean, μ, is located just to the right of the peak.
   

References

Data from Parade Magazine.

“HIV/AIDS Epidemiology Santa Clara County.”Santa Clara County Public Health Department, May 2011.

Concept Review

The chi-square distribution is a useful tool for assessment in a series of problem categories. These problem categories include primarily (i) whether a data set fits a particular distribution, (ii) whether the distributions of two populations are the same, (iii) whether two events might be independent, and (iv) whether there is a different variability than expected within a population.

An important parameter in a chi-square distribution is the degrees of freedom df in a given problem. The random variable in the chi-square distribution is the sum of squares of df standard normal variables, which must be independent. The key characteristics of the chi-square distribution also depend directly on the degrees of freedom.

The chi-square distribution curve is skewed to the right, and its shape depends on the degrees of freedom df. For df > 90, the curve approximates the normal distribution. Test statistics based on the chi-square distribution are always greater than or equal to zero. Such application tests are almost always right-tailed tests.

Formula Review

$displaystylech{i}^2=(Z_1{)}^2+(Z_2{)}^2+dots(Z_{df}{)}^2$ chi-square distribution random variable

$displaystylem{u}_{ch{i}^2}=df$ chi-square distribution population mean

$displaystylesigm{a}_{ch{i}^2}=sqrt2(df)$ Chi-Square distribution population standard deviation